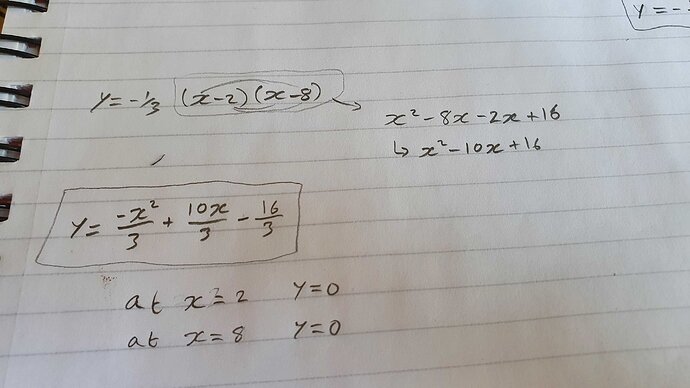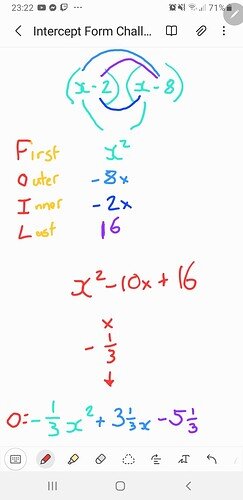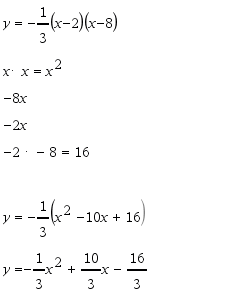In this lecture we learnt about the FOIL (First-Outer-Inner-Last) method, which can be used to convert our quadratic equations from intercept form to standard form.
For your challenge, convert the following equation into standard form;
y = -1/3(x - 2)(x - 8)
Post your answer below and remember to use spoiler tags.
y = -(1/3)(x - 2)(x - 8)
y = -(1/3)(x² - 8x - 2x + 16)
y = -(1/3)x² + (10/3)x - (16/3)
I’m not quite clear on the FOIL method. I did my studies in Italy, and this seems like it was just the standard way of multiplying binomials. Is this basically just a mnemonic tool for how to multiply binomials or it it something more?
Oh yeah, here’s my answer:
y = (-x^2 + 10x -16)/3 I’m imagining this as a long fraction line under all the terms with the 3 underneath (looks better on paper than on screen)
@olidadda, FOIL is just a mnemonic for First-Outer-Inner-Last - so the order in which you do the expansion.
“The FOIL method” just sounds a little less scary than “multiplying binomials” and the term is commonly used in the UK and US (and probably some other places too) when introducing the concept.
So there’s nothing deeper going on, its just a way of making things slightly easier to digest.
perfect, I’m sort of glad that’s all there is to it 
I got:
0 = -1/3x^2 + 10/3x - 16/3x
Ah - it should be + 16/3, correct? I forgot to cancel out the negative.
@Ajai_Raj, you were correct with the -16/3 but you had a rogue ‘x’ at the end 
Not sure if you picked it up from somewhere by mistake or if it was just a typo.
Whoops, yeah that was a typo
y=−(1/3)(x−2)(x−8)
y=−(1/3)(x^2−8x−2x+16)
y=−(1/3) x^2+(10/3)x −(16/3)
y = -1/3(x-2)(x-8)
(x-2)(x-8)
x2 -8x -2x + 16
(x2 -10x + 16) * -1/3
-1/3x2 + 10/3x - 16/3
y = -1/3x2 + 10/3x - 16/3
I was doing this along with your example while watching the video so didn’t have a challenge to do at the end haha!
And another round:
0 = -1/3(x - 2)(x - 8)
x * x = x²
x * (-8) = -8x
(-2) * x = -2x
(-2) * (-8) = 16
-1/3(x² - 10x +16)
0 = -1/3x² + 10/3x - 16/3
Here is mine:
0 = -1/3x^2 + 10/3x - 16/3
y=(-1/3)x^2+(10/3)-(16/3)
