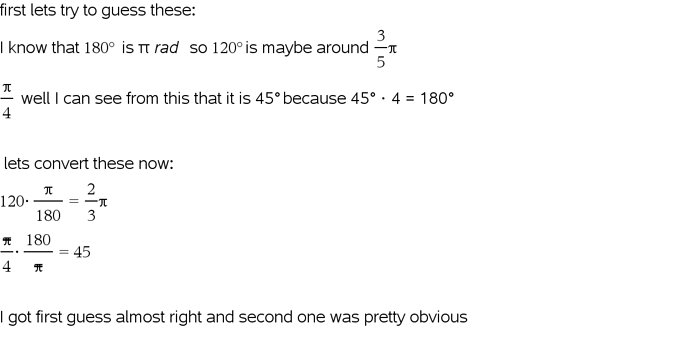Math - Radians and Degrees - Challenge

Other Courses Talk
,
#1

In this lecture we looked at how measure an angle using either degrees or radians. We also worked out how to quickly convert between the two.

For your challenge, I’d like you to;

  1. Convert 120 degrees into radians - and you can give your answers in terms of either π (Pi) or τ (Tau).

  2. Convert τ/8 (which is π/4) and convert it back in to degrees.

To help build your intuition when dealing with radians, also try to guess the answers before you actually solve them for real - Were you close?

Post your answers below and remember to use the spoiler tags.

#2

Challenge Accepted!

1. I got T/3 . Tau divided by three.

Hey Gary, is question number two supposed to be asking to convert to degrees? Because it says convert it back to radians but it is already a radian.

2. I got 45 degrees

I love the idea of building your intuition. I love the unit circle and even though it’s been awhile since I’ve used one I had a good idea what the answer would be. I love these challenges!

1 Like
#3

1 : tau/3 or 2/3 * pi or 2.1
2 : 45

PS i think the diagram at the begining of the lecture could be clearer when explaining radians that it’s the arc that is also one in length as well as the radius. It is mentioned but showing one on the diagram could make it clearer if that bit is missed.

1 Like
#4

@Kevin-Brandon, good spot. I’ve fixed the wording of the question - part 2 should be to convert radians to degrees :slight_smile:

Thank you for the feedback @EddieRocks, I’ll review the lecture and see if I can make this clearer.
The arc is drawn onto the circumference when I explain it, but orange on green probably isn’t the best color combination for making it easy to see!

2 Likes
#5
  1. 120° = 2.09 Rad
  2. Pi/8 = 22.5° This is easy because Pi/2 is 90°
1 Like
#6

@olidadda,
The first one is correct, but what is the answer in terms of π or τ?
The second one is right but not for the question - the question was for τ/8 rather than π/8.

You’re on the right lines thinking about the problem in terms of fractions of a circle though.
Using your example, if τ is twice as big as π then; 90° = 1/4 circle = τ/4 = π/2.
So you should be able to use the same logic to get both answers.

1 Like
#7

120° to RAD --> 120 * PI / 180 = 2.0944
PI / 4 to DEG --> PI / 4 * 180 / PI = 45°

1 Like
#8

Guessed: ~2 rad
Answer: (tau/360) x 120 = 120(tau)/360 = 1/3(tau) = ~2.093 rad

Guessed: 45 degrees
Answer: 360/tau * tau/8 = 360/8 = 45 degrees

1 Like
#9

Nice work @ajai.

A good way to thing about the first one it as fractions of a circle.
So if 120 degrees is 1/3 of a circle, then this will be τ/3 radians - You’re just adding tau to the top of your fraction. Likewise, if you had 3/4 of a circle, this would be 3τ/4 and so on.

Guessing 2 rad is still a pretty good estimate though, so well done :slight_smile:

3 Likes
#10

1). Guess : Since 120° is 1/3 of a circle then my guess would be τ/3
Math:
τ/360∗120=120/360 τ=1/3 τ or τ/3

2). Guess: since it’s τ/8 then perhaps 1/8 of a circle or 360/8=45
Math:
360/τ∗τ/8=360/8=45

1 Like
#11

1. 120° is 2/3 Pi or Tau/3
2. Tau/8 or Pi/4 is 45°

#12

Problem 1:

120 Degrees to Radians

(π/180) * a

(π/180) * 120

2.094 Radians

Problem 2:

π/4 to degrees

π/4* 180/π = θ

θ = 45 Degrees

1 Like
#13

1) τ/3
2) 45 degrees

1 Like
#14

Here are my results:

120° * 𝛕/360° = 𝛉
𝛉 = 𝛕/3
𝛉 = 2,09

I guessed 45° beforehand.
360°/𝛕 * 𝛕/8 = 𝛉
𝛉 = 360°/8
𝛉 = 45°

Checkm8!

1 Like
#15

Here is mine:

image
image1251×616 16.2 KB

1 Like
#16

I wasn’t sure what to guess for 120 degrees, but i got 2Pi/3 when calculating

I guessed 45 degrees for Pi/4, and calculated 45 degrees.

1 Like
#17

120 to rads = 2.09
t/8 or pi/4 = 45

1 Like
#18
  1. 2 * pi / 3 or tau / 3
  2. 45°
#19
  1. Convert 120 degrees into radians - and you can give your answers in terms of either π (Pi) or τ (Tau).
    Answer : 2/3 π or 1/3 Tau
  2. Convert τ/8 (which is π/4) and convert it back in to degrees.
    Answer : 1/8 of a circle or 45 degrees.
#20

  1. 120° * (pi / 180rad) = 2.094 rad
    2 * pi / 3

  2. pi/4 = 0.785
    0.785 rad * 180/pi = 45°

Privacy & Terms