Using our Volcano scenario again:
y = -1/3x^2 + 10/3 - 16/3
Convert to intercept form using the Add and Multiply method:
-3y = x^2 - 10x + 16
(5 + u)(5 - u) = 16
25 -u^2 = 16
u^2 = 25 - 16
u^2 = 9
u = 3
(5 + 3)(5 - 3) = 16
8 x 2 = 16
8 + 2 = 10
y = -1/3(x - 8)(x - 2)
Roots = (2,0) (8,0)
How do we know to put a plus or a minus in the brackets. You say in the video Im using negatives in here so I’m looking for a the positive version. But it left me not knowing really if it was us that was deciding it and if we wanted we could change these?
Also when we have for example:
(3 + u)(3 - u) = 14
is the negative in
9 - u = 14
Because the + and the - in the above multiplication create a negative?
Sorry for the simple questions, but Im not good at maths hence the maths course.
Cheers
Phew, been a while since I’ve posted on this course! Here’s what I’ve done for the challenge:
y = 1/3x^2 + 10/3x - 16/3
-3y = x^2 - 10x + 16
-3y = (x-8)(x-2)
y = -1/3(x-8)(x-2)
Seems like this is quite a common answer so hoping it’s correct!
i get
y=-1/3 (x-8) (x-2)
y=-(1/3)x^2+(10/3)x-16/3 =>
-3y=x^2-10x+16 (where (5-u)(5+u)=16 => 25-u^2=16 => u^2=9 => u=+3 or -3) =>
-3y=(x-2)*(x-8) => y=-(1/3)(x-2)(x-8)
y = -1/3 x^2 + 10/3 x - 16/3
-3y = x^2 - 10x + 16
(5 - u) * (5 + u) = 16
25 - u^2 = 16
u^2 = 25 - 16
u^2 = 9
u = √9
u = 3
-3y = (x - 2) * (x - 8)
y = -1/3 (x - 2) (x - 8)
y = -1/3x^2 + 10/3x - 16/3
-3y = x^2 - 10x + 16
(5 + u)(5 - u) = 16
25 - u^2 = 16
u^2 = 9
u = 3
(5 +3)(5 - 3) = 16
8 * 2 =16
-3y = (x - 2)(x -8)
y = -1/3(x - 2)(x - 8)
This is more my speed. 
y = (-1/3)x^2 + (10/3)x - (16/3)
(R) = -3
-3y = x^2 - (30/3)x + (48/3)
-3y = x^2 - 10x + 16
(5+u)(5-u) = 16
25
-5u
5u
-u^2
u^2 = 25 - 16
u^2 = 9
u = sqr(9)
u = 3
(5+3)(5-3) = 5
(8)(2) = 5
8+2 == 10
8*2 == 16
Haha, I got mine completely wrong.
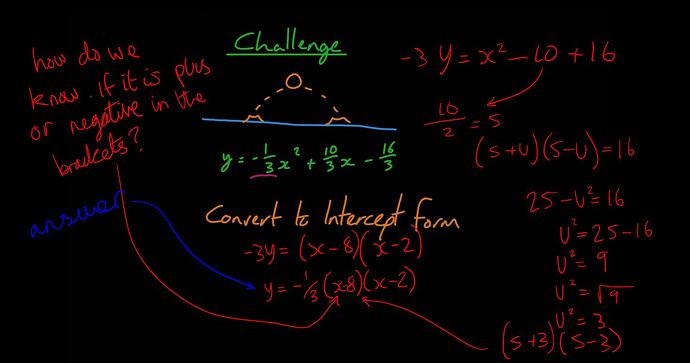
Here’s my answer for the Factoring Quadratics challenge:
Q1. Given y = (-1/3)x² + (10/3)x - (16/3), convert to vertex form
A1. y = (-1/3)(x - 2)(x - 8)
A1a. y = (-1/3)x² + (10/3)x - (16/3)
A1b -3y = x² - 10x + 16
Divide b by two to get 5, insert into equation below and solve:
A1c.(5 + u)(5 - u) = 16
A1d. 25 - u² = 16
A1fe -u² = -9
A1f. u² = 9
A1g. u = 3
Insert result of 3 back into original and solve:
A1h. (5 + 3)(5 - 3) = 16
A1i. 8 * 2 = 16
This means our two roots are (2, 0) and (8, 0). We can now insert these values into our intercept form equation for p and q as well as our already known value for a. This gives us:
y = (-1/3)(x - 2)(x - 8)
Convert to intercept form (find the roots) of y = -1/3x^2 + 10/3x - 16/3
Overall it went well. I’m starting to get all of the things mixed up
